The algebraic sum of the products of the currents by the respective resistance around a closed loop is equal to the algebraic sum of the emf´s in the loop.
Note: An emf is considered positive IF the arbitrary direction around the loop coincides with the direction of the emf of the current source. The figure bellow gives an example to the formula application.
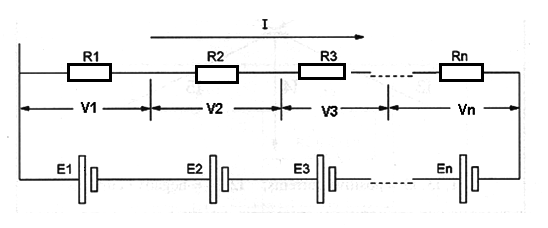
Formula 1
E1 + E2 + E3 + .... + En = V1 + V2 + V3 + .... + Vn
Where:
E1, E2, E3 ... En are the emf in volts (V)
V1, V2, V3...Vn are the voltage falls accross the resistors R1, R2, R3...Rn in volts (V)
Derivated Formula:
Formula 2
E1 + E2 + E3 +.... + Em = R1 x I + R2 x I + R3 x I + ..... + Rn X I
Where:
R1, R2, R3 ... Rn are the resistances of the resistors in the loop in ohm (Ω)
E1, E2, E3... En are the emf in volts (V)
I is the current flowing through the loop in amperes (A)




